knion
Member
- Dec 29, 2020
- 37
Hey!
(As this is its own thread, I'd like to say that a lot of this first part is in relation to previous posts I have made on this website. If you want to read the actual helpful information, I would scroll to the end!
And, yet again, I'm sorry for posting so much).
FIRSTLY I WANT TO SAY THAT I HAVE MADE A GRAVE MISTAKE IN MY ORIGINAL POST.
I WILL KEEP IT POSTED AS A LOT OF THE INFORMATION (such as pictures and the weights of carbon) MAY STILL BE USEFUL.
HOWEVER, MY CALCULATIONS OF THE SPACE WITHIN A CAR AND THE TIME IT WOULD TAKE TO FILL WAS WRONG.
I HAVE NOW FIXED THIS MISTAKE IN THIS COMMENT/ POST.
THESE CALCULATIONS ARE NOT ONLY MORE ACCURATE BECAUSE I DID NOT MAKE A STUPID MISTAKE, BUT THEY ARE ALSO MUCH MUCH MORE IN DEPTH.
I would like to thank Bedrock48 for their comment. Without it I wouldn't have found my mistake.
I also realise that I am posting a lot on this website, so don't worry, I will take a while off after this! Haha.
IF YOU WISH TO SEE THE HELPFUL STUFF, RATHER THAN A WHOLE LOT OF WORKINGS OUT, PLEASE SCROLL STRAIGHT TO THE BOTTOM.
Ok, so it took a little while, but I found some info on this.
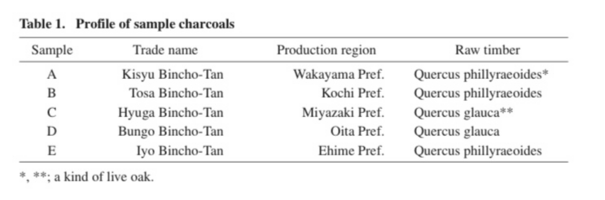
Here is a picture that outlines the properties of different types of charcoal (incase anyone was interested)

I also found a study about the concentration of CO in a certain area. And this study appears to have used Binchotan as it's charcoal of choice.
"if the concentration of carbon in the briquettes is 85%, the lethal concentration of CO is 10 000 ppm and the volume of an apartment is 100 m3, then burning 629 g of charcoal briquettes could produce a potentially toxic atmosphere." - (https://www.mja.com.au/journal/2012...nduced-death-and-toxicity-charcoal-briquettes).
Sadly this study didn't focus on the time it took to produce this effect.
HOWEVER, it does tell us that only 629 g of Binchotan charcoal briquettes will achieve 10000ppm at some point, a particularly lethal concentration.
To help understand what this means and even try to figure out the timings on this, I also found another study.
This study measured the speed at which 50-100g of charcoal produced Carbon monoxide.
these were the results:
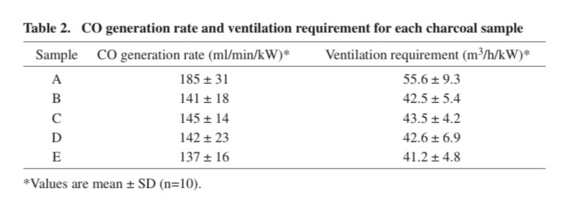
(https://www.jniosh.johas.go.jp/en/indu_hel/doc/IH_49_3_393.pdf)
I'm entirely new to the "±" symbol, but apparently it is "the confidence interval or error in a measurement"
So, for example, sample A, where it says 185 ± 31, it actually just means *roughly 185*. This is because, if I'm right, the outcome is within the realm of 185 but potentially plus OR minus 31.
It's at this point I'd like to say that I'm sorry. This reply is extremely lengthy.
I at least hope that they are genuinely useful.
Anyways.
To continue, it looks as though (If I understand this correctly), the 'best' binchotan (sample A) which i'd imagine is 85% carbon at least, releases roughly 185 ml of CO per minute.
To figure out how long it would take to get to a high enough ppm using this 85% carbon, we'll need to do some equations.
By the way, for anyone confused, as I was for a long while, PPM is an abbreviation for "parts per million" and it also can be expressed as milligrams per liter (mg/L)
PPM is calculated by dividing the mass of the solute by the mass of the solution, then multiplying by 1,000,000
So.
Let's use the example of the the room from earlier (100 m3). This room as litres would be 100,000 litres
1,000 ml is a litre.
185 ml is 0.185 litres.
So, the mass of the 'solution' (the air in the room), is 100,000 litres.
(1 minute of carbon burning) 0.185(ml) X 60 (mins) = 11.1 (litres)
11.1 (litres) / 100,000 (litres) = 0.000111 (litres)
0.000111 (litres) X 1,000,000 = 111 ppm
So it would take an hour for 50-100g (let's say 75g) of 85% Carbon to get to 111pm in a 100m3 room.
I mean, this obviously isn't going to be enough to satisfyingly end your life. So, let's use the amount of charcoal from earlier.
75g/75 = 1g
111pm / 75 = 1.48
1.48 X 629 = 930.92
So, 629 grams of 85% charcoal in a 100m3 room would take an hour to get to 930.92 ppm of CO.
So this means, in the initial test that I talked about, they probably measured that it got to 10,000 ppm after around, well, 10 hours
(which would obviously still make sense and be alarming in the context of the research, because it is surrounding cooking inside buildings such as restaurants, which are open for extended periods of time)
Anyway.
To finally answer your (Bedrock48) question.
If we assume that binchotan is 85% charcoal, and the BBQ charcoal briquettes are roughly 75% you would be correct in saying that the effect would not only create less smoke (as that is something binchotan does), but it would also cause death much more quickly, (or you would at least need more of the briquettes to achieve the same effect, but not A LOT more as far as I can tell).
If we want prove this, all we really need to do is as follows:
If it takes 629 grams of 85% charcoal an hour to fill a 100m3 room with 930.92 ppm, then:
629g / 85 = 7.4 g
85g - 75g = 10g
85g + 10g = 95
7.4 X 95 = 703
It would take 704 grams of 75% carbon an hour to fill a 100m3 room with 930.92 ppm.
So 85% charcoal only needs 629 grams to beat 75% charcoal's 704 grams to achieve the same goal in the same time.
CHARCOAL CARBON MONOXIDE IN A SMALL SPACE.
So, as I said at the beginning of this post/ reply, I made a massive mistake in the original post.
So, this is where I would like to make up my mistake by talking about the time it would take to die in the same space as, say, a car, through this method.
(Just an FYI, it gets even MORE tricky from this point.)
So, 930.92 ppm is 930.92 g/ litre.
A single m3 is 1000 litres and 100m3 holds 100,000 litres.
So If it takes one hour to reach 930.92 g/litre in a 100,000 litre room, Then, in a room only 3,000 litres big:
100/3 = 33.43333333333333
33.43333333333333 X 1,000 = 33,433.33333
33,433.33333 X 930.92 = 31,123,758.66666667
An hour would've been enough time to reach 31,123,758.666666667ppm (which obviously doesn't make sense).
So this means, if 60 minutes would have had to have passed to achieve the 31,123,758.7ppm, we would achieve 1ppm in 0.000001927787728 minutes. This is 1.927788e seconds
SO, to achieve the lethal dose of 10,000ppm that we want in our (on average) 3 cubic metre car, we would want to wait:
1ppm X 10,000 = 10,000 ppm
1.927788e X 10,000 = 19,277.88
This is equal to 5.3547222222222 hours!
(And this is the case with 705 grams of 75% charcoal, and 629 grams of 85% charcoal, more charcoal would achieve affects more quickly)
ANYWAYS, THE REAL IMPORTANT, MINI VERSION OF THIS STUFF IS BELOW:
If you saw this post/ reply and thought, wow, that's way too much to read. Here is what I have found.
It would take 629 grams of 85% charcoal (binchotan) one hour to reach 930.92 ppm in a room 100 cubic metres big.
It would take 704 grams of 75% charcoal to have the same effect.
AND, most importantly, it would take just over 5 hours for 629 grams of 85% charcoal, OR 704 grams of 75% charcoal to reach 10,000ppm in a car with an interior that measures 3 cubic.
Anyways.
Yet again, I profusely apologise for the mistake I made in my last post. I am extremely sorry about that.
I hope that this message will be helpful and easy to read for all of you who wish to CTB by carbon monoxide poisoning via Charcoal.
Thank you all so much,
Knion.
(As this is its own thread, I'd like to say that a lot of this first part is in relation to previous posts I have made on this website. If you want to read the actual helpful information, I would scroll to the end!
And, yet again, I'm sorry for posting so much).
FIRSTLY I WANT TO SAY THAT I HAVE MADE A GRAVE MISTAKE IN MY ORIGINAL POST.
I WILL KEEP IT POSTED AS A LOT OF THE INFORMATION (such as pictures and the weights of carbon) MAY STILL BE USEFUL.
HOWEVER, MY CALCULATIONS OF THE SPACE WITHIN A CAR AND THE TIME IT WOULD TAKE TO FILL WAS WRONG.
I HAVE NOW FIXED THIS MISTAKE IN THIS COMMENT/ POST.
THESE CALCULATIONS ARE NOT ONLY MORE ACCURATE BECAUSE I DID NOT MAKE A STUPID MISTAKE, BUT THEY ARE ALSO MUCH MUCH MORE IN DEPTH.
I would like to thank Bedrock48 for their comment. Without it I wouldn't have found my mistake.
I also realise that I am posting a lot on this website, so don't worry, I will take a while off after this! Haha.
IF YOU WISH TO SEE THE HELPFUL STUFF, RATHER THAN A WHOLE LOT OF WORKINGS OUT, PLEASE SCROLL STRAIGHT TO THE BOTTOM.
Ok, so it took a little while, but I found some info on this.
Here is a picture that outlines the properties of different types of charcoal (incase anyone was interested)

I also found a study about the concentration of CO in a certain area. And this study appears to have used Binchotan as it's charcoal of choice.
"if the concentration of carbon in the briquettes is 85%, the lethal concentration of CO is 10 000 ppm and the volume of an apartment is 100 m3, then burning 629 g of charcoal briquettes could produce a potentially toxic atmosphere." - (https://www.mja.com.au/journal/2012...nduced-death-and-toxicity-charcoal-briquettes).
Sadly this study didn't focus on the time it took to produce this effect.
HOWEVER, it does tell us that only 629 g of Binchotan charcoal briquettes will achieve 10000ppm at some point, a particularly lethal concentration.
To help understand what this means and even try to figure out the timings on this, I also found another study.
This study measured the speed at which 50-100g of charcoal produced Carbon monoxide.
these were the results:


(https://www.jniosh.johas.go.jp/en/indu_hel/doc/IH_49_3_393.pdf)
I'm entirely new to the "±" symbol, but apparently it is "the confidence interval or error in a measurement"
So, for example, sample A, where it says 185 ± 31, it actually just means *roughly 185*. This is because, if I'm right, the outcome is within the realm of 185 but potentially plus OR minus 31.
It's at this point I'd like to say that I'm sorry. This reply is extremely lengthy.
I at least hope that they are genuinely useful.
Anyways.
To continue, it looks as though (If I understand this correctly), the 'best' binchotan (sample A) which i'd imagine is 85% carbon at least, releases roughly 185 ml of CO per minute.
To figure out how long it would take to get to a high enough ppm using this 85% carbon, we'll need to do some equations.
By the way, for anyone confused, as I was for a long while, PPM is an abbreviation for "parts per million" and it also can be expressed as milligrams per liter (mg/L)
PPM is calculated by dividing the mass of the solute by the mass of the solution, then multiplying by 1,000,000
So.
Let's use the example of the the room from earlier (100 m3). This room as litres would be 100,000 litres
1,000 ml is a litre.
185 ml is 0.185 litres.
So, the mass of the 'solution' (the air in the room), is 100,000 litres.
(1 minute of carbon burning) 0.185(ml) X 60 (mins) = 11.1 (litres)
11.1 (litres) / 100,000 (litres) = 0.000111 (litres)
0.000111 (litres) X 1,000,000 = 111 ppm
So it would take an hour for 50-100g (let's say 75g) of 85% Carbon to get to 111pm in a 100m3 room.
I mean, this obviously isn't going to be enough to satisfyingly end your life. So, let's use the amount of charcoal from earlier.
75g/75 = 1g
111pm / 75 = 1.48
1.48 X 629 = 930.92
So, 629 grams of 85% charcoal in a 100m3 room would take an hour to get to 930.92 ppm of CO.
So this means, in the initial test that I talked about, they probably measured that it got to 10,000 ppm after around, well, 10 hours
(which would obviously still make sense and be alarming in the context of the research, because it is surrounding cooking inside buildings such as restaurants, which are open for extended periods of time)
Anyway.
To finally answer your (Bedrock48) question.
If we assume that binchotan is 85% charcoal, and the BBQ charcoal briquettes are roughly 75% you would be correct in saying that the effect would not only create less smoke (as that is something binchotan does), but it would also cause death much more quickly, (or you would at least need more of the briquettes to achieve the same effect, but not A LOT more as far as I can tell).
If we want prove this, all we really need to do is as follows:
If it takes 629 grams of 85% charcoal an hour to fill a 100m3 room with 930.92 ppm, then:
629g / 85 = 7.4 g
85g - 75g = 10g
85g + 10g = 95
7.4 X 95 = 703
It would take 704 grams of 75% carbon an hour to fill a 100m3 room with 930.92 ppm.
So 85% charcoal only needs 629 grams to beat 75% charcoal's 704 grams to achieve the same goal in the same time.
CHARCOAL CARBON MONOXIDE IN A SMALL SPACE.
So, as I said at the beginning of this post/ reply, I made a massive mistake in the original post.
So, this is where I would like to make up my mistake by talking about the time it would take to die in the same space as, say, a car, through this method.
(Just an FYI, it gets even MORE tricky from this point.)
So, 930.92 ppm is 930.92 g/ litre.
A single m3 is 1000 litres and 100m3 holds 100,000 litres.
So If it takes one hour to reach 930.92 g/litre in a 100,000 litre room, Then, in a room only 3,000 litres big:
100/3 = 33.43333333333333
33.43333333333333 X 1,000 = 33,433.33333
33,433.33333 X 930.92 = 31,123,758.66666667
An hour would've been enough time to reach 31,123,758.666666667ppm (which obviously doesn't make sense).
So this means, if 60 minutes would have had to have passed to achieve the 31,123,758.7ppm, we would achieve 1ppm in 0.000001927787728 minutes. This is 1.927788e seconds
SO, to achieve the lethal dose of 10,000ppm that we want in our (on average) 3 cubic metre car, we would want to wait:
1ppm X 10,000 = 10,000 ppm
1.927788e X 10,000 = 19,277.88
This is equal to 5.3547222222222 hours!
(And this is the case with 705 grams of 75% charcoal, and 629 grams of 85% charcoal, more charcoal would achieve affects more quickly)
ANYWAYS, THE REAL IMPORTANT, MINI VERSION OF THIS STUFF IS BELOW:
If you saw this post/ reply and thought, wow, that's way too much to read. Here is what I have found.
| 1,600 ppm (0.16%), (1.6‰) | Headache, increased heart rate, dizziness, and nausea within 20 min; death in less than 2 hour |
| 3,200 ppm (0.32%), (3.2‰) | Headache, dizziness and nausea in five to ten minutes. Death within 30 mins. |
| 6,400 ppm (0.64%), (6.4‰) | Headache and dizziness in one to two minutes. Convulsions, respiratory arrest, and death in less than 20 minutes |
| 12,800 ppm (1.28%), (12.8‰) | Unconsciousness after 2–3 breaths. Death in less than three minutes |
It would take 629 grams of 85% charcoal (binchotan) one hour to reach 930.92 ppm in a room 100 cubic metres big.
It would take 704 grams of 75% charcoal to have the same effect.
AND, most importantly, it would take just over 5 hours for 629 grams of 85% charcoal, OR 704 grams of 75% charcoal to reach 10,000ppm in a car with an interior that measures 3 cubic.
Anyways.
Yet again, I profusely apologise for the mistake I made in my last post. I am extremely sorry about that.
I hope that this message will be helpful and easy to read for all of you who wish to CTB by carbon monoxide poisoning via Charcoal.
Thank you all so much,
Knion.